Go to the top page

多面体おりがみ

英	日
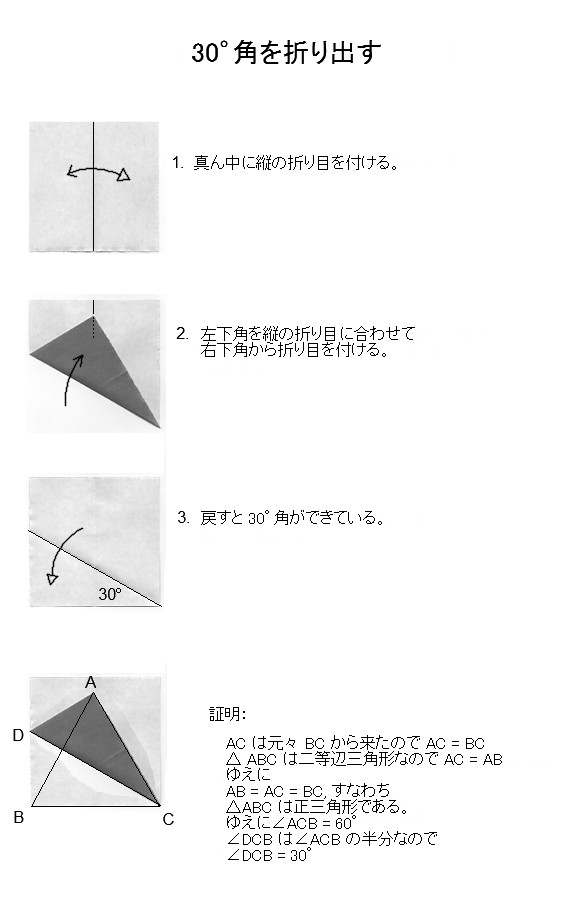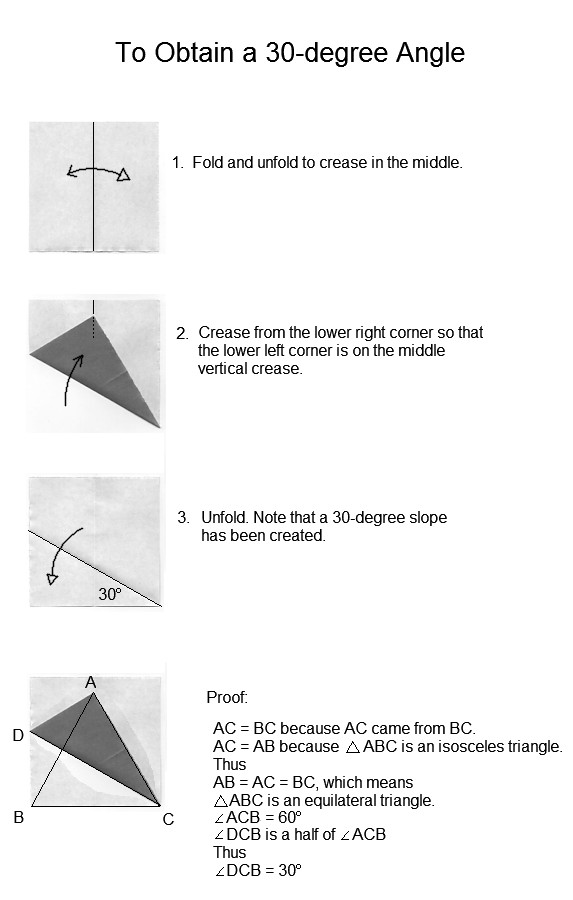
To Obtain a 30-degree Angle	30°角を折り出す
1. Fold and unfold to crease in the middle.	1. 真ん中に縦の折り目を付ける。
2. Crease from the lower right corner so that the lower left corner is on the middle vertical crease.	2. 左下角を縦の折り目に合わせて右下角から折り目を付ける。
3. Unfold. Note that a 30-degree slope has been created.	3. 戻すと 30°角ができている。
Proof:	証明:
AC = BC because AC came from BC. AC = AB because △ABC is an isosceles triangle. Thus AB = AC = BC, which means △ABC is an equilateral triangle. ∠ACB = 60° ∠DCB is a half of ∠ACB Thus ∠DCB = 30°	AC は元々 BC から来たので AC = BC △ABC は二等辺三角形なので AC = AB ゆえに AB = AC = BC すなわち △ABC は正三角形である。 ゆえに ∠ACB = 60° ∠DCB は ∠ACB の半分なので ∠DCB = 30°

（Both English and Japanese parts have been authored by Osamu Takeuchi.)